$\theta+\dfrac{\pi}{2}$の三角関数
$\theta+\dfrac{\pi}{2}$の三角関数
暗記$\theta+\dfrac{\pi}{2}$の三角関数
$\sin\left(\theta+\dfrac{\pi}{2}\right),\cos\left(\theta+\dfrac{\pi}{2}\right),\tan\left(\theta+\dfrac{\pi}{2}\right)$を,それぞれ$\sin\theta,\cos\theta,\tan\theta$で表せ.
無題
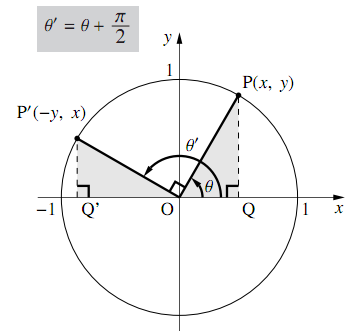
図のように,単位円周上に角$\theta$の動径$\text{OP}$と 角$\theta+\dfrac{\pi}{2}$($ = \theta'$とする)の動径$\text{OP}'$をとる.
点$\text{P}$の座標を$(x,~y)$とすると,$ \triangle{\text{OPQ}}と\triangle{\text{OP}'\text{Q}'}$は合同なので,点$\text{P}'$の座標は$(-y,~x)$となるから
\begin{align} &\sin{\theta'}=x=\boldsymbol{\cos\theta}\\ &\cos{\theta'}=-y=\boldsymbol{-\sin\theta}\\ &\tan{\theta'}=\dfrac{x}{-y}=\boldsymbol{-\dfrac{1}{\tan\theta}} \end{align}$\theta+\dfrac{\pi}{2}$の三角比
無題
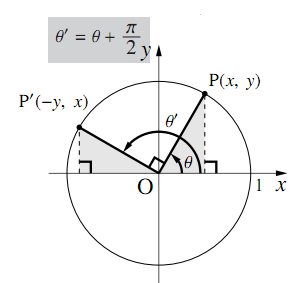
任意の角$\theta$について
\begin{align} &\sin\left(\theta+\dfrac{\pi}{2}\right)=\cos\theta\\ &\cos\left(\theta+\dfrac{\pi}{2}\right)=-\sin\theta\\ &\tan\left(\theta+\dfrac{\pi}{2}\right)=-\dfrac{1}{\tan\theta} \end{align}が成り立つ.
吹き出し$\theta+\dfrac{\pi}{2}$の三角関数
この節で学んだ公式は丸暗記するようなものではない. 図を書いてすぐに導けるように練習しておこう.